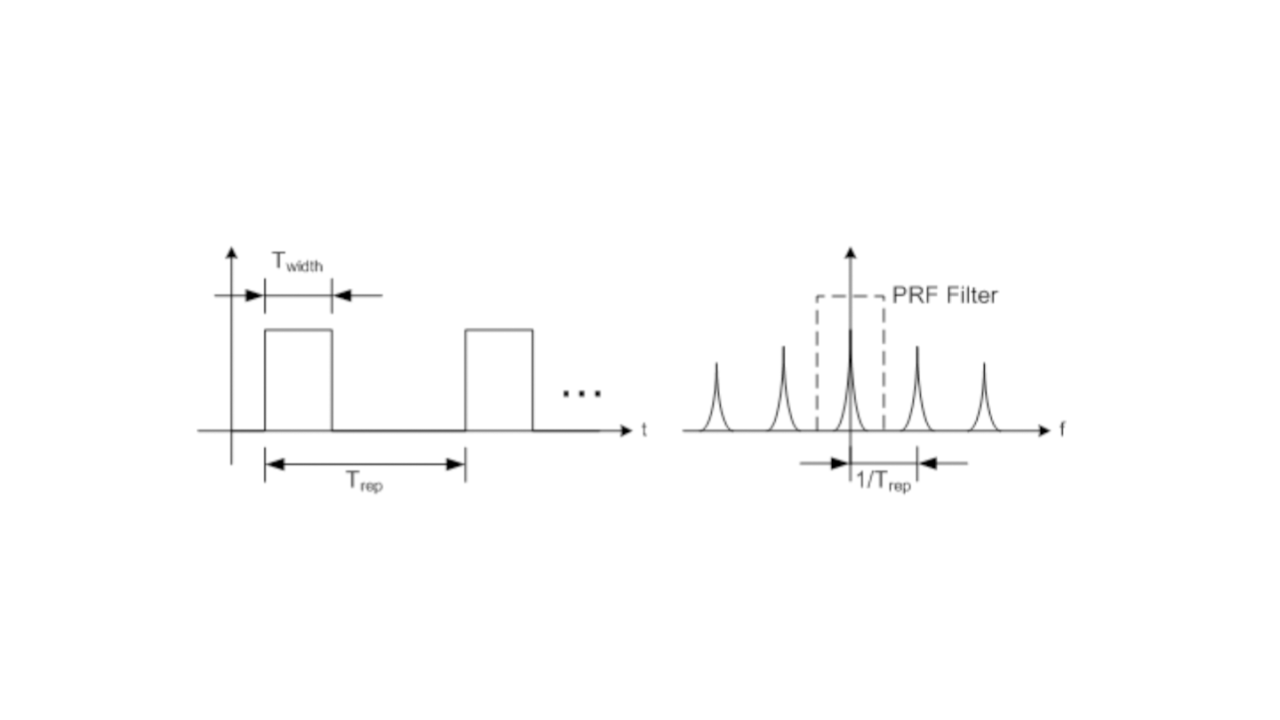
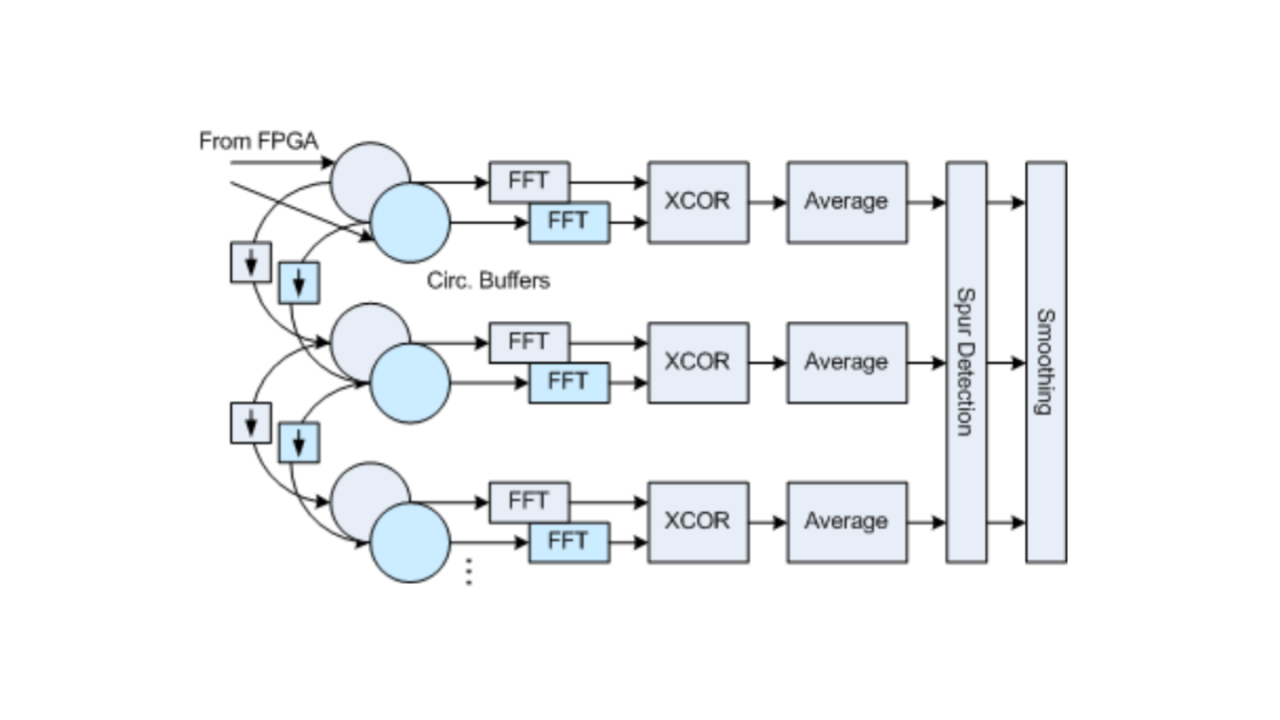
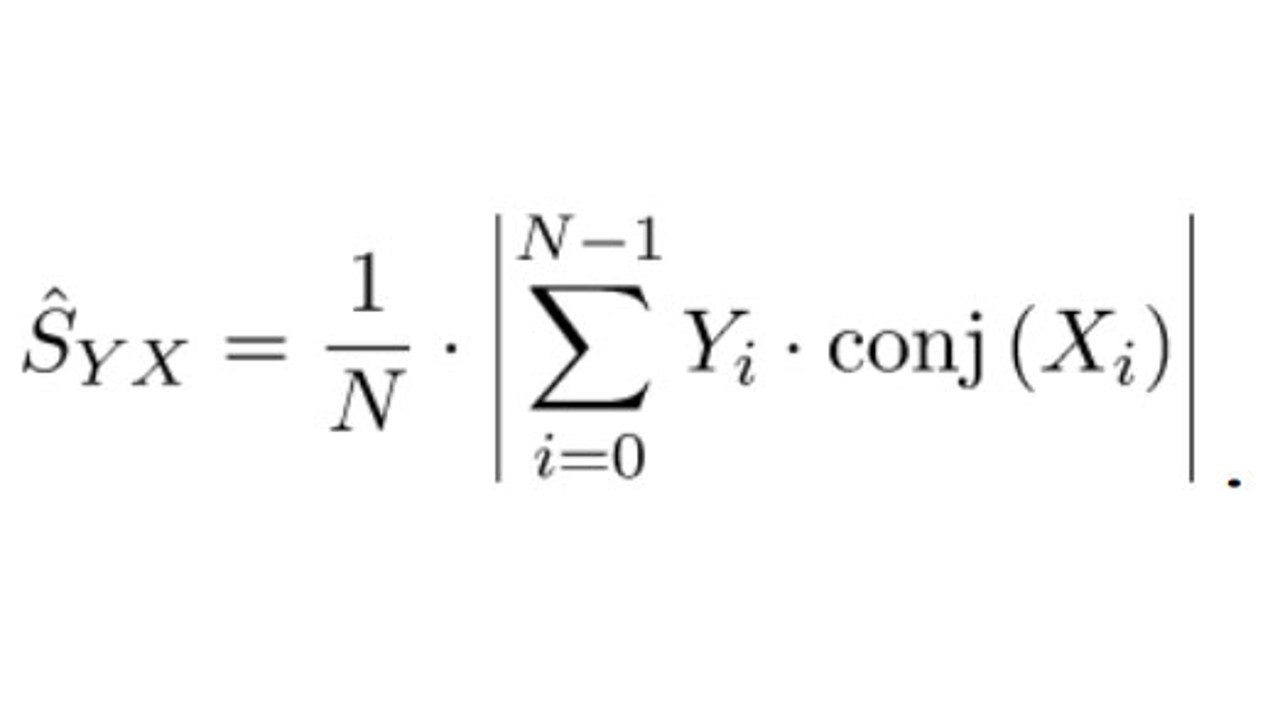A 1 MHz to 50 GHz Direct Down-Conversion Phase Noise Analyzer with Cross-Correlation
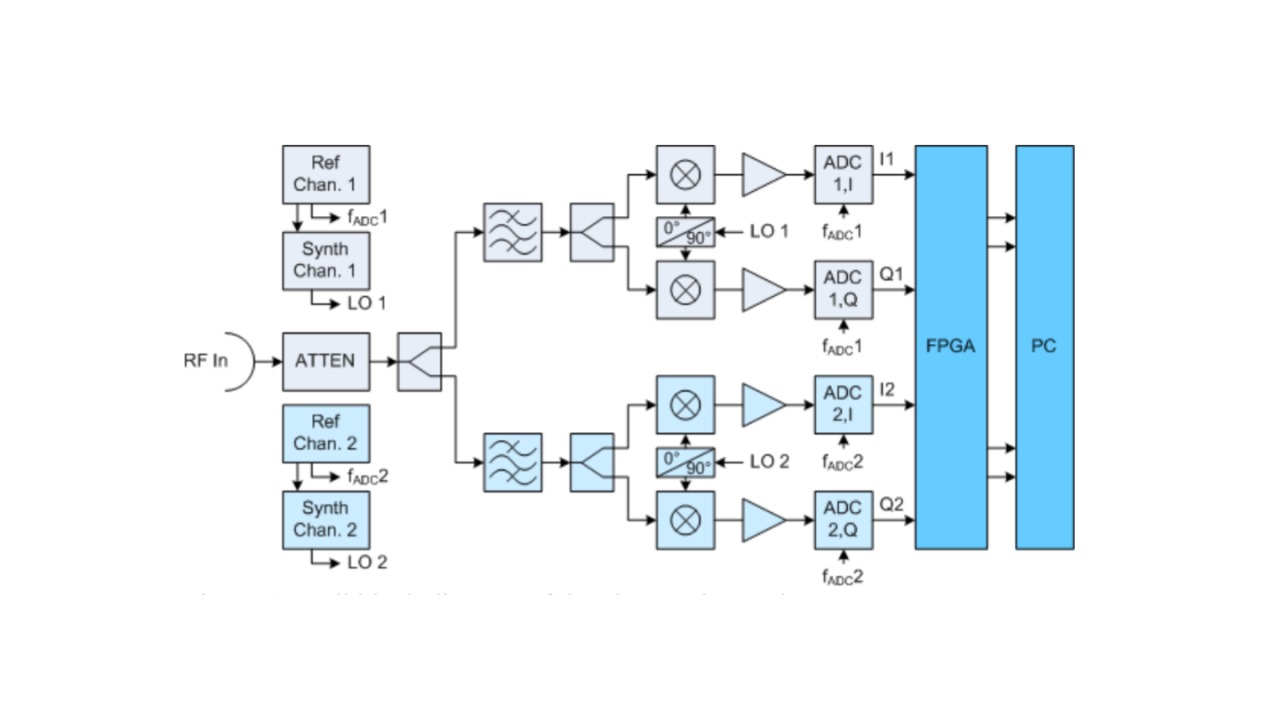
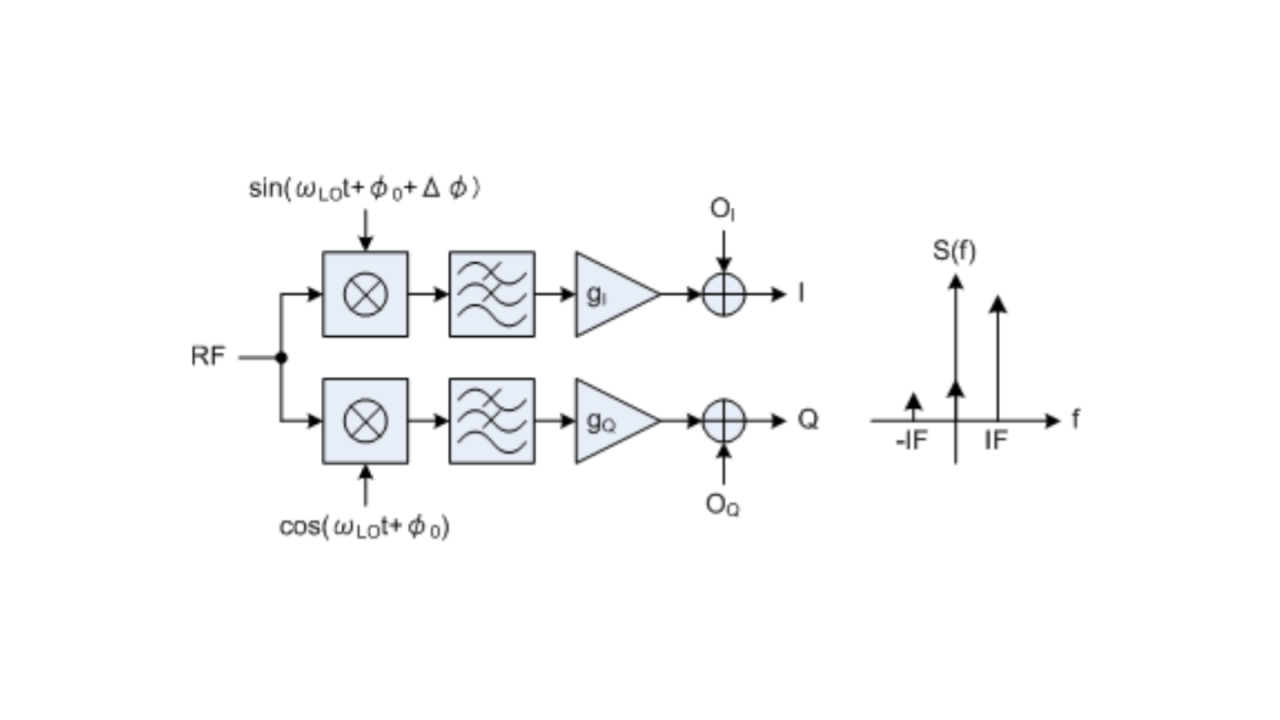
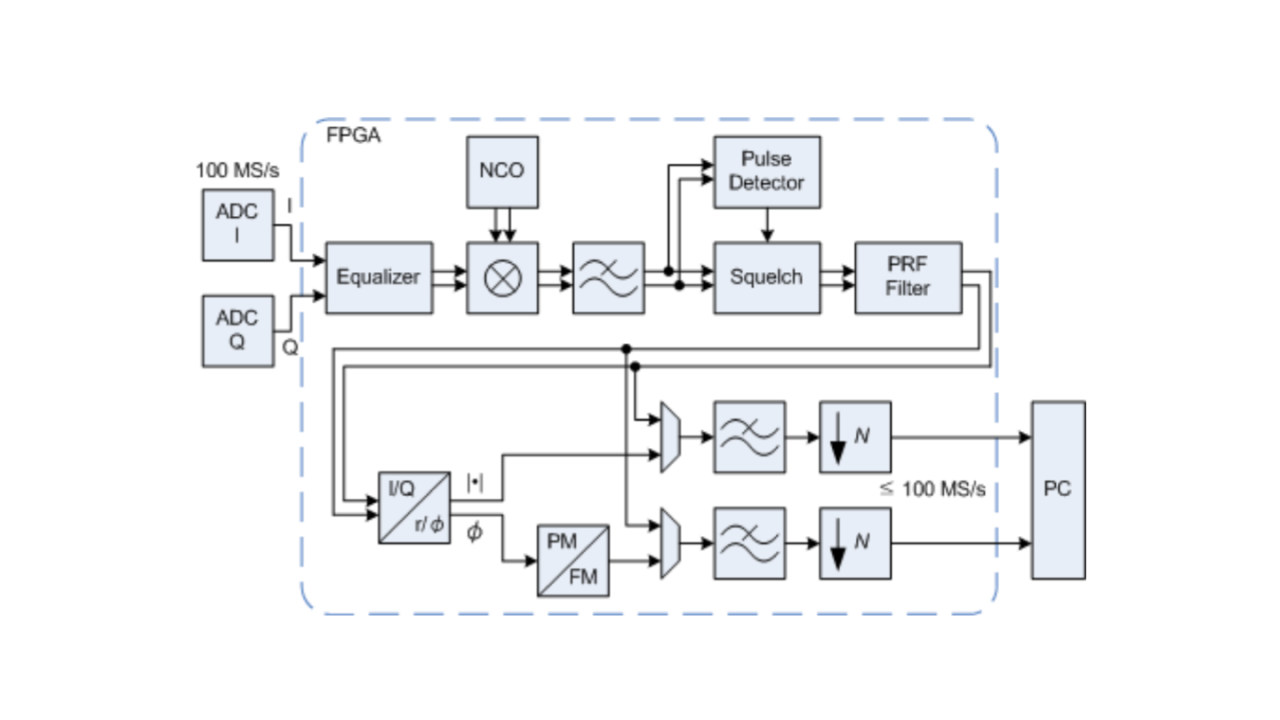
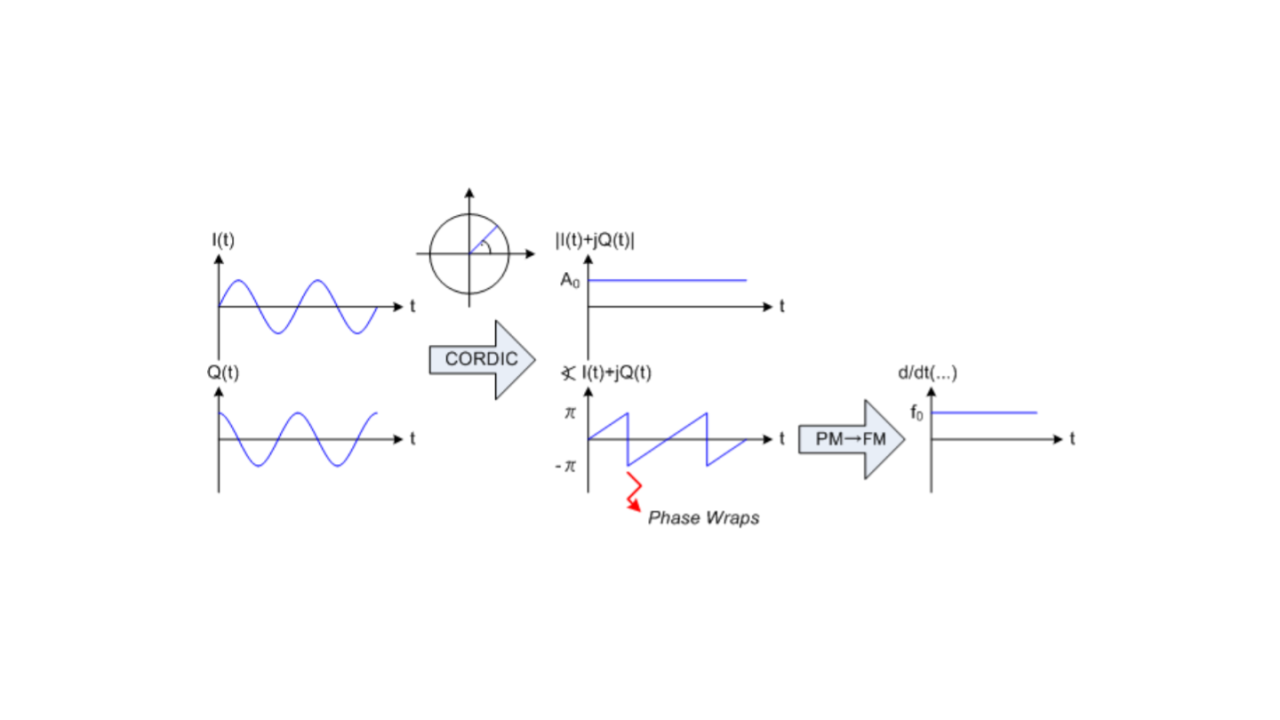
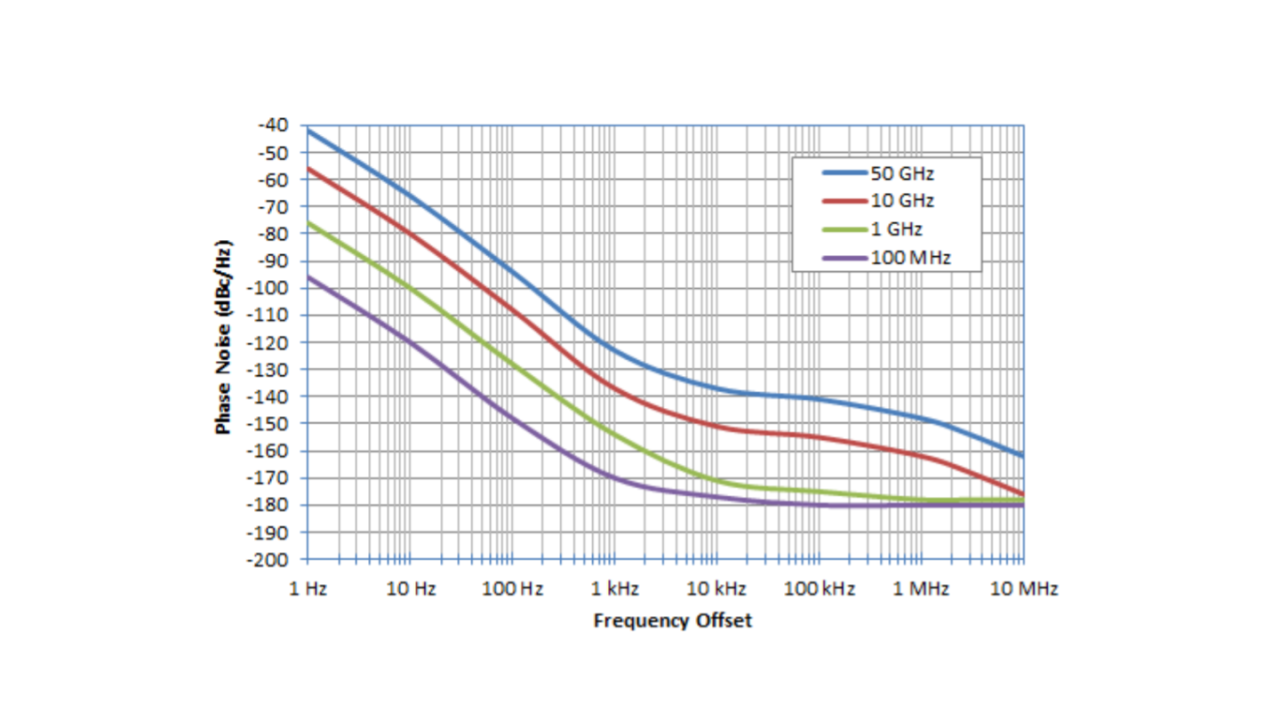
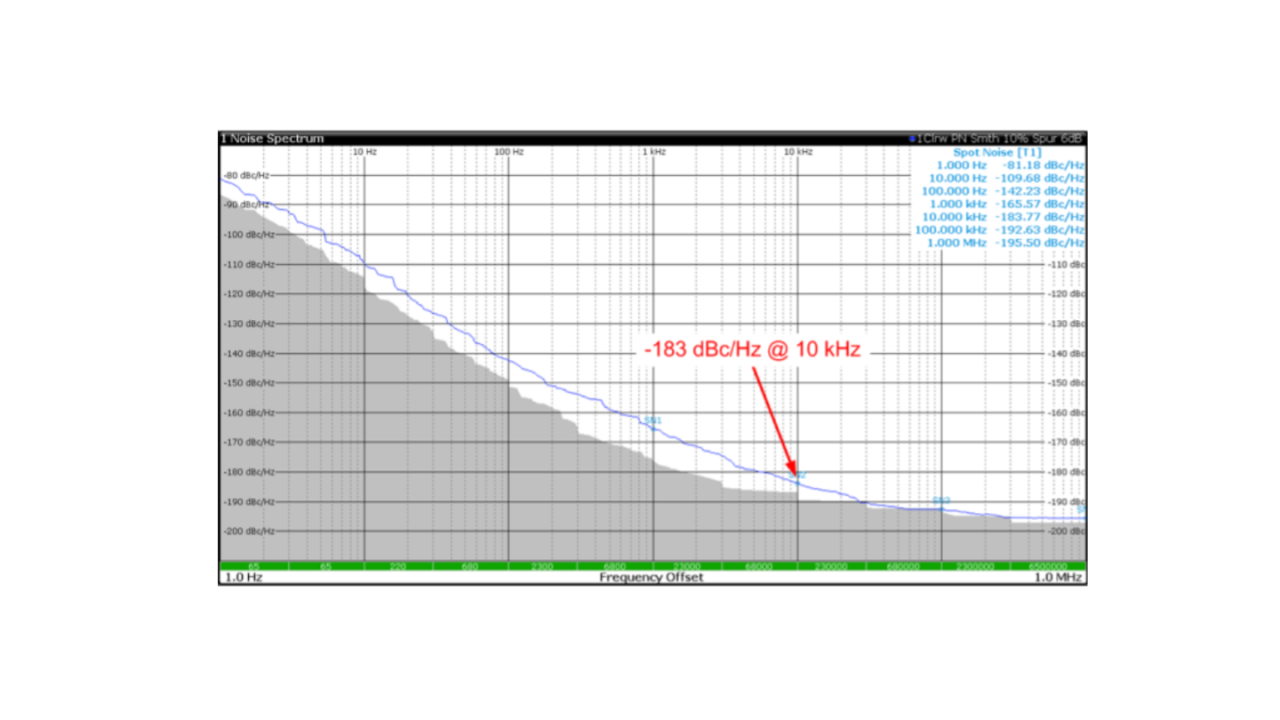
A new phase noise test instrument covers the frequency range from 1 MHz to 50 GHz with direct down-conversion analog I/Q mixers and baseband signal sampling. The traditional PLL has been replaced by a digital FM demodulator for phase detection and frequency tracking. An additional AM demodulator enables concurrent measurement of phase and amplitude noise. The instrument can measure phase noise as low as -183 dBc/Hz with a 100 MHz carrier frequency and 10 kHz offset within two minutes.